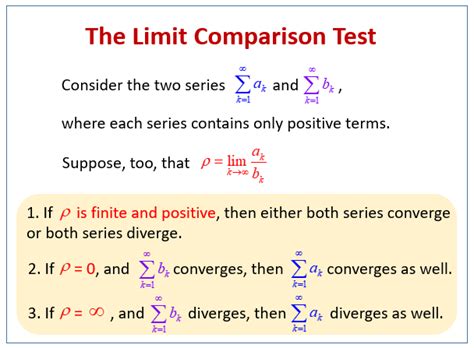
limit comparison test hard questions|Calculus II : fabrication The Limit Comparison Test: Suppose an > 0 and bn > 0 for all n. If lim. n→∞. the two series X .
This not only enables the user to forcibly remove air by using a vacuum on the chamber before a cycle (known as pre-vacuum), but it also enables them to use a vacuum after the cycle (known as post-vacuum) to .
{plog:ftitle_list}
Discover the ultimate guide to steam (autoclave) sterilization for healthcare professionals. Learn the principles, processes, and critical role in patient care.In central processing, double wrapping can be done sequentially or nonsequentially (i.e., simultaneous wrapping). Wrapping should be done in such a manner to avoid tenting and gapping. The sequential wrap uses two sheets of the standard sterilization .
Here is a set of practice problems to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University.10.6 Integral Test; 10.7 Comparison Test/Limit Comparison Test; 10.8 .
Here is a set of assignement problems (for use by instructors) to accompany the .In this section we will discuss using the Comparison Test and Limit Comparison . In this section we will discuss using the Comparison Test and Limit Comparison .
The Limit Comparison Test: Suppose an > 0 and bn > 0 for all n. If lim. n→∞. the two series X . This section explains the Direct and Limit Comparison Tests for determining the .
Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 .
The Limit Comparison Test. an. Suppose an > 0 and bn > 0 for all n. If lim. n!1. = c, where c is .Evaluate the Direct Comparison Test and the Limit Comparison Test in determining the .How to use the limit comparison test to determine whether or not a given series converges or .
for all integers n ≥ 2. Although we could look for a different series with which to compare ∞ ∑ n .
The limit comparison test - Ximera. We compare infinite series to each other using limits. Using . Here is a set of practice problems to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University. In this section we will discuss using the Comparison Test and Limit Comparison Tests to determine if an infinite series converges or diverges. In order to use either test the terms of the infinite series must be positive. Proofs for both tests are also given.The Limit Comparison Test: Suppose an > 0 and bn > 0 for all n. If lim. n→∞. the two series X an and X bn either both converge or both diverge. ∞. 1. Example 1: Determine whether the series. converges or diverges. 2n + n.
This section explains the Direct and Limit Comparison Tests for determining the convergence or divergence of series. The Direct Comparison Test involves comparing terms with a known series, while the .
Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 converges or diverges. 27) ∞ ∑ n = 1(1 − 1 n)n. n (Hint: (1 − 1 n)n → 1 / e.)The Limit Comparison Test. an. Suppose an > 0 and bn > 0 for all n. If lim. n!1. = c, where c is bn series P an and P bn either both converge or both diverge. nite and c > 0, then the two. owing series can be proven to converge or diverge by comparing to a kno.Evaluate the Direct Comparison Test and the Limit Comparison Test in determining the convergence or divergence of series with positive terms. Discuss the limitations and advantages of each test, providing insights into their practical implications.
How to use the limit comparison test to determine whether or not a given series converges or diverges, examples and step by step solutions, A series of free online calculus lectures in videos
for all integers n ≥ 2. Although we could look for a different series with which to compare ∞ ∑ n = 2 1 (n2 − 1), instead we show how we can use the limit comparison test to compare. ∞ ∑ n = 2 1 n2 − 1 and ∞ ∑ n = 2 1 n2. Let us examine the idea behind the limit comparison test.
The limit comparison test - Ximera. We compare infinite series to each other using limits. Using the comparison test can be hard, because finding the right sequence of inequalities is difficult. The limit comparison test eliminates this part of the method. Here is a set of practice problems to accompany the Comparison Test/Limit Comparison Test section of the Series & Sequences chapter of the notes for Paul Dawkins Calculus II course at Lamar University. In this section we will discuss using the Comparison Test and Limit Comparison Tests to determine if an infinite series converges or diverges. In order to use either test the terms of the infinite series must be positive. Proofs for both tests are also given.
The Limit Comparison Test: Suppose an > 0 and bn > 0 for all n. If lim. n→∞. the two series X an and X bn either both converge or both diverge. ∞. 1. Example 1: Determine whether the series. converges or diverges. 2n + n. This section explains the Direct and Limit Comparison Tests for determining the convergence or divergence of series. The Direct Comparison Test involves comparing terms with a known series, while the .Use the Limit Comparison Test to determine whether each series in exercises 14 - 28 converges or diverges. 27) ∞ ∑ n = 1(1 − 1 n)n. n (Hint: (1 − 1 n)n → 1 / e.)
The Limit Comparison Test. an. Suppose an > 0 and bn > 0 for all n. If lim. n!1. = c, where c is bn series P an and P bn either both converge or both diverge. nite and c > 0, then the two. owing series can be proven to converge or diverge by comparing to a kno.Evaluate the Direct Comparison Test and the Limit Comparison Test in determining the convergence or divergence of series with positive terms. Discuss the limitations and advantages of each test, providing insights into their practical implications.How to use the limit comparison test to determine whether or not a given series converges or diverges, examples and step by step solutions, A series of free online calculus lectures in videos
The limit comparison test
for all integers n ≥ 2. Although we could look for a different series with which to compare ∞ ∑ n = 2 1 (n2 − 1), instead we show how we can use the limit comparison test to compare. ∞ ∑ n = 2 1 n2 − 1 and ∞ ∑ n = 2 1 n2. Let us examine the idea behind the limit comparison test.
.jpg)
centrifugal solar pump
digital prp centrifuge machine
As the autoclave infrastructure in the world’s many healthcare institutions, learning laboratories and research facilities continue to age — the issue of sterilizing staining will undoubtedly continue to arise. Contact CSSif you’re experiencing staining issues and we’ll put you in touch with one of our autoclave experts. See more
limit comparison test hard questions|Calculus II